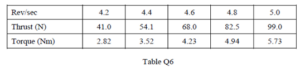DieselShip's Online Content Library

Q1. The load water plane of a ship 120 m long, floating In Sea water of density 1025 kg/m³, is defined by the half ordinates given in Table Q1:

The following particulars are obtained from the ship's hydrostatic curves:

displacement = 8450 tonne

centre of buoyancy above the keel (KB) = 3.21 m

moment to change trim by one centimetre (MCT lcm) = 101.5 tm

Calculate EACH of the following:

(a) the position of the longitudinal centre Of flotation (LCF) from midships; (6)

(b) the second moment of area of the waterptane about a transverse axis through the centroid; (6)

(c) the height Of the ship's centre Of gravity above the keel (KG). (4)

Q3. A Ship Of length 130 m has a light displacement Of 4800 tonne with the longitudinal centre of gravity 0.5 m aft of midships. Loading now takes place as given in Table Q3.

Table Q3

Using Worksheet Q3, extract the relevant data from the hydrostatic curves and hence determine the final end draughts of the vessel in sea water of density 1025 kg/ m³. (16)

Q4. A ship of displacement 11000 tonne has a length 120 m and an even keel draught of 5.5 m in sea water of density 1025 kg/m³.

The area of the waterplane is 1440 m² and the second moment of area of the waterptane about a transverse axis through midships is 1.2 x 106 m4 with the LCF at midships. The ship has a full depth empty rectangular compartment having a length 12 m and breadth 10 m. The centre of the compartment is on the centretine of the ship 40 m forward of midships. Calculate the end draughts after the compartment is bilged.

Note: For the purposes of calculating the MCT1cm it can be assumed that GML= BML. (16)

Q5. The hull of a box shaped vessel is 80 m long and has a mass of 800 tonne uniformly distributed over its length. Machinery of mass 200 tonne extends uniformly over the middle 20 m length of the vessel.

Two holds extending over the extreme forward and aft 20 m lengths of the vessel, each have 240 tonne of cargo stowed uniformly over their lengths.

(a) Construct curves of EACH of the following:

(i) load per metre; (8)

(ii) shearing force. (4)

(b) Calculate the value of the maximum bending moment. (4)

Q6. The force acting normal to the plane of a rudder is given by the expression:

Fn = 20.2 A V² a newtons

Where :         A = rudder area (m²)

V = ship speed (m/s)

a = rudder angle (degrees)

A manoeuvrability specification for a ship that requires a transverse rudder force of 92 kN is generated when the angle of helm is 35^o with the ship travelling at 5 knots.

(a) Determine suitable dimensions for a rectangular rudder having an aspect ratio (depth to width ratio) of 1.5.  (6)

(b) The rudder stock is designed to have a diameter of 360 mm with the allowable shear stress in the material limited to 70 MN/m² at its service speed of 16 knots. At the maximum helm angle of 35^o, the centre of effort is 35% of the rudder width from the leading edge of the rudder.

Calculate the maximum distance of the axis of rotation from the leading edge of the rudder so that the stock is not overstressed at the service speed. (10)

Q7. (a) State TWO of the MINOR components of the residuary resistance.  (2)

(b) A ship has a length of 140 m and floats in sea water of density 1025 kg/³. A geometrically similar model of this ship has a length of 5 m and a wetted surface area of 5.8 m².  The model has a total resistance of 29.55 N when towed in fresh water of density 1000 kg/m³ at a speed corresponding to 15 knots for the ship. Calculate EACH of the following:

(i) the ratio of residuary resistance to total resistance for the model at the corresponding speed; (6)

(ii) the ratio of residuary resistance to total resistance for the ship. (6)

(c) State why the TWO ratios calculated in Q7(b) should be different.  (2)

Note:  The frictional coefficient the model in fresh water is 1.694

The frictional coefficient for the ship in sea water is 1.415

Speed in rms with the speed index (n) for ship and model 1.825

Q8. A model propeller of 0.4 m diameter and 0.95 pitch ratio, is tested under conditions of constant speed of advance of 1.25 m/s in fresh water of density 1000 kg/m³.

The thrust and torque values for a range of rotational speeds are given in Table Q8.

(a) Draw Curves Of propeller efficiency and delivered power against real slip ratio for the model propeller.  (9)

(b) Using the curves drawn in Q8(a), determine EACH Of the following:

(i) the model propeller rotational speed for maximum efficiency; (4)

(ii) the delivered for a geometrically Similar ships propeller of 6.5 m diameter, operating in sea water of density 1025 kg/m³ at a real slip ratio of 0.3, given that delivered power is proportional to (diameter)^3.5 (3)

Q9. (a) Show that the position of the centre of pressure for a rectangular plane, with its edge in surface, is two thirds of the depth of the plane below the surface.  (3)

(b) A dock gate 12 m wide and 8 m has river water of density 1008 kg/m3 on one side to a depth of 3 m and sea water of 1025 kg/m³ on the other side to a depth of 5 m.

Calculate EACH Of the following:

(i) the resultant load; (6)

(ii) the position of the centre of pressure. (4)

(c) The depth of on the river water side of the gate is increased until the load on each side of the gate is equal. Calculate this new depth of water of river water. (3)